Hallo,
Ik heb een vraag over de volgende opdracht:
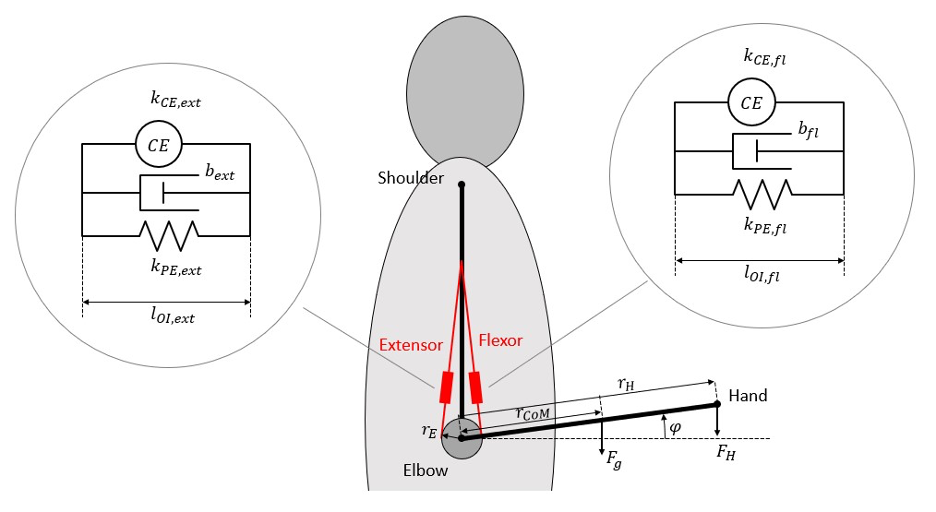
The presented modified muscle model can be used to calculate muscle forces. The structure of the model is the same for the flexor and extensor. Express the muscle force in the flexor as a function of the active state (𝑞𝑓𝑙), the elbow joint angle (𝜑), and the elbow joint angular velocity (𝜑̇). Assume that the passive elements do not generate any force when the elbow is in 90° of flexion (as shown in Figure 1). Note: The relationship between the arc length 𝑙 of a circle, it’s radius 𝑟, and angle 𝜑 is described by: 𝑙 = 𝜑 ∙ 𝑟 .
Kunt u mij op weg helpen? Ik weet niet goed hoe ik de variabelen zou moeten implementeren in een functie.
Alvast bedankt,
Roos